Finite orbits

Suppose that $E/F$ is an algebraic field extension, and that $F=E^G$ for some subgroup of automorphisms of $E$ (that is, the extension is Galois). Show that if $e\in E$ then the orbit of $e$ under $\mathrm{Gal}(E/F)$ must be finite. Show that $G$ need not be finite, and show that this fails if the extension is not assumed to be algebraic.

One thought on “Finite orbits”

Since the action of $\mathrm{Gal}(E/F)$ fixes $F$ , it must permute the roots of the minimal polynomial of $e$ over $F$ , which exists since the extension is algebraic, so the cardinality of its orbit is bounded by the degree of this polynomial.

As for the second part, take any infinite Galois extension (for instance $\overline{\Bbb{Q}}/\Bbb{Q}$ ).

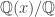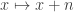For the last question, if the extension is not algebraic, the orbit of an element by the action of the Galois group may not be finite. For instance, consider $\Bbb{Q}(x)/\Bbb{Q}$ with $x$ trascendental over $\Bbb{Q}$ . Then the $\Bbb{Q}$ -algebra maps $x\mapsto x+n$ are in the Galois group and so the orbit of $x$ contains $x+n$ for all $n\in\Bbb{Z}$ .